The second step in Fenton-like chemistry in water: calculation of the free energy barrier of O-O homolysis of Fe(III)OOH

One of the proposed second reaction steps in the oxidation catalysis by the Fenton-like reagent involves homolysis of the oxygen-oxygen bond of the iron(III)hydro-peroxo species, producing an OH. radical and the ferryl ion (reaction 6.5). We have studied this reaction in detail, since it has emerged from our static DFT calculations as one of the most likely second reaction steps, in particular when the hydrolysis of a water ligand is simultaneously taken into account.

We have performed constrained AIMD simulations to calculate the free energy profile for the oxygen-oxygen bond homolysis reaction of the [Fe $^{\rm {III}}$ (H

OOH] $^{2+}$ complex into an iron(IV)oxo species and an OH. radical in water. The oxygen-oxygen bond length $R_{\rm {OO}}$ was taken as the constrained reaction coordinate, which seems intuitively a good choice that includes the most important contribution to the intrinsic reaction coordinate. The main drawback of this choice is however that it does not prevent unwanted side reactions such as the abstraction of solvent molecule hydrogens by the OH. radical produced. For large values of the constrained reaction coordinate $R_{\rm {OO}}$ , the OH. radical can abstract a solvent hydrogen forming H

O, while an OH. species "jumps" through the solvent by a chain reaction. The sampled force of constraint, associated with the force necessary to keep a H

O molecule (instead of the OH.) constrained to the oxygen of the iron complex, will then be of course meaningless. We will therefore take the same approach as was done in the work by Trout and Parrinello, who studied the dissociation of H

O in water in H

and OH

with the same technique,[207] and only calculated the profile up to (or at least very close to) the transition state. Since the subsequent reaction of the OH. "jumping" into the solvent is thermoneutral, the free energy profile of the homolysis is not expected to decrease by more than a few kcal/mol beyond the transition state (due to the increasing entropy of the leaving OH. radical and the solvation of the oxo site). Moreover, the transition state energy is the more important parameter to determine whether the oxygen-oxygen homolysis is indeed a probable mechanism.

**Figure 6.4:** The mean force of constraint $\left <F \right >_{R}$ (dashed line; right-hand-side axis) and the Helmholtz free energy $\Delta A$ (solid line; left-hand-side axis) versus the oxygen-oxygen distance $R_{\rm{OO}}$ . The crosses denote the values from constrained MD runs sampled during 2 picoseconds and the circles denote the average constraint force during 1.3 ps, up to the moment that the OH. radical abstracts a hydrogen from a nearby solvent molecule. The dotted lines give the energies of the hydrated complex in vacuo, calculated with the ADF program (triangles) and with the PAW program (squares). The limits of infinitely far separated products (reaction I in table 6.1) are indicated by vertical dashed lines.

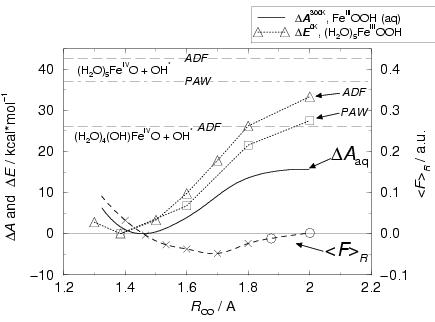
Eight constrained AIMD runs were performed with constrained oxygen-oxygen bond lengths varying from $R_{\rm {OO}}=1.4 - 2.0$ Å. The initial configuration of each constrained simulation was taken from the last frame of the first simulation of hydroperoxo iron(III) in water, including the hydronium ion (see section 6.3.2). For each system, a short AIMD simulation was started to bring $R_{\rm {OO}}$ to the desired value in 2000 steps. Then equilibration of each system took place for 2 picoseconds, after which the force of constraint was accumulated for another 2 picoseconds. The obtained values for the mean force of constraint are denoted by crosses and circles in figure 6.4 and fitted with a quadratic spline. Integration of the mean force of constraint gives the Helmholtz free energy profile $\Delta A(R_{\rm {OO}})$ , where we take the minimum at

Å for the offset of the energy scale (solid line). The circles indicate those constrained simulations during which the O $^\beta$ H. radical abstracts a hydrogen and transforms into a water molecule. Indeed, this occurs for the $R_{\rm {OO}}$ values close to the transition state, for which the O $^\beta$ H part has acquired enough radical character to abstract a hydrogen when a nearby solvent molecule moves into a suitable position. In both cases (at $R_{\rm {OO}}=1.875$ Å and at $R_{\rm {OO}}=2.000$ Å), the hydrogen abstraction occurs after about 1.3 ps simulation. The values for the mean force of constraint denoted by the circles are the averages over these 1.3 ps. After the H abstraction by O $^\beta$ H., the force of constraint goes to zero, or becomes even slightly positive (repulsive) because the produced water molecule is repelled by the oxo ligand at the short constrained oxygen-oxygen distance, rather than attracted like the OH. radical shortly before. For the O-O distance of $R_{\rm {OO}}=2.000$ Å, the average force of constraint (over the 1.3 ps before the H abstraction) is almost equal to zero, which indicates that this O-O distance is indeed very close to the transition state.

The free energy reaction barrier for the homolysis reaction in water is found to be $\Delta A^{\ddagger}=15.7$ kcal/mol, which is low compared to the $\Delta E=42.6$ kcal/mol (ground-state) energy change found for the reaction in vacuo, or even the $\Delta E=26.1$ kcal/mol for the hydrolyzed complexes in vacuo (reaction I in table 6.1). We have also plotted twice the contour for the reaction energy $\Delta E^{0\rm {K}}$ of the homolysis of the [Fe $^{\rm {III}}$ (H

OOH] $^{2+}$ complex in vacuo (triangles connected by a dotted line, and the value for infinite product separation indicated by the vertical dashed line); once computed with the ADF program and once computed with the PAW program. Unfortunately, we find an increasing underestimation of the energy profile with increasing $R_{\rm {OO}}$ , calculated with PAW compared to the highly accurate (all-electron, large basis set) ADF results, with a maximum difference of 5.6 kcal/mol at $R_{\rm {OO}}=2.0$ Å and at infinite separation. The error does not seem to be due to the plane-wave cutoff of 30 Ry (it is only reduced by 0.5 kcal/mol when going to 50 Ry) and can be attributed to the partial waves for the inner region of the valence electrons and the projector functions for the iron atom used in the PAW calculations. Although bond energies in iron(III) and iron(IV) complexes computed with PAW agree within 2 kcal/mol with those using ADF, we have found after extensive tests that the stability of the (formally) Fe $^{4+}$ configuration is overestimated by 5-6 kcal/mol with respect to the (formally) Fe $^{3+}$ configuration. This indicates that also the free energy barrier of the homolysis in aqueous solution has to be corrected for this error so that the true value becomes $\Delta A^{\ddagger}\approx 21$ kcal/mol. Solvent effects thus strongly reduce the transition state barrier for the O-O homolysis reaction in water. The main contribution to this effect is expected to originate from the larger absolute energy of solvation for the separating transition state complex (Fe $^\mathrm{IV}$ =O $\cdots$ OH.) in comparison with the reactant molecule, Fe $^\mathrm{III}$ OOH. (Note that often reaction barriers are increased in aqueous solution, because the sum of the absolute energies of solvation for two reacting molecules is typically larger than that for the single transition state complex.)

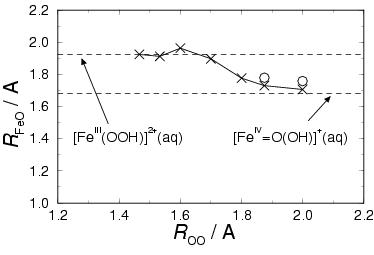
The upper graph in figure 6.5 illustrates the transformation of the Fe $^{\rm {III}}$ -OOH

bond into an Fe $^{\rm {IV}}$ =O $^{2-}$ bond by showing the average Fe-O $^\alpha$ distance as a function of the reaction coordinate. The two dashed lines indicate the average $R_{\rm {FeO}}$ for the pentaaquairon(III)hydroperoxo complex in water (obtained from the first 5 ps simulation described in section 6.3.2), equal to 1.922 Å and the average $R_{\rm {FeO}}$ for the ferryl ion in water equal to 1.680 Å. This latter number was obtained from the [Fe $^{\rm {IV}}$ O(OH)]

moiety produced in the reaction between Fe $^{\rm {II}}$ and H

in water (cf. ref bernd4), in which indeed a water ligand hydrolyzed to form the OH

ligand as suggested in section 6.3.1. This apparent increased acidity of the iron(IV) species compared to that of iron(III) is discussed below. Proceeding with the Fe-O distance, we see that $R_{\rm {FeO}}$ decreases rapidly when the oxygen-oxygen separation becomes larger than 1.7 Å, which indicates the changing character of the metal and the bonds. At the reaction coordinate value of $R_{\rm {OO}}=2.0$ Å, the average $R_{\rm {FeO}}$ (over the 1.3 ps simulation before the O $^\beta$ H. radical abstracts a solvent hydrogen) has decreased practically to the average value of free [Fe $^{\rm {IV}}$ O(OH)]

, which indicates that at $R_{\rm {OO}}=2.0$ Å the reaction is close to completion. The open circles in figure 6.5 denote the averages over the simulation part after the O $^\beta$ H. radical transformed into H

O $^\beta$ by H-abstraction from an adjacent solvent molecule. At $R_{\rm {OO}}=1.6$ Å, the average $R_{\rm {FeO}}$ is a little larger than expected from the trend. This is the result of proton donation from the iron complex (i.e. hydrolysis) to the aqueous solvent during the constrained simulation at this reaction coordinate value, which we will explain below.

**Figure 6.5:** Left-hand-side graph: average Fe-O $\alpha$ bond length as a function of the constrained reaction coordinate $R_{OO}$ . Two dashed lines indicate the average values for reactant and product iron complexes in solution, respectively. Open circles denote the average $R_{FeO}$ after the product O $^\beta$ H. transformed into HO by H-abstraction from an adjacent solvent molecule. Right-hand-side graph: average number of hydronium ions as a function of the reaction coordinate.

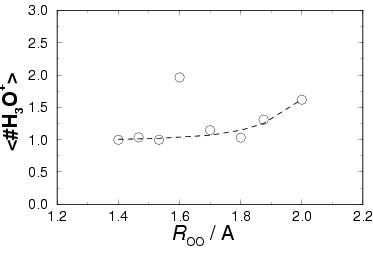
As mentioned before, we expect hydrolysis of water ligands to lower the reaction energy of the oxygen-oxygen homolysis (see the change from 42.6 to 26.1 kcal/mol for reaction I in table 6.1), and secondly we expect hydrolysis to become more frequent for iron(IV) (product) compared to iron(III) (reactant). In our short constrained dynamics simulations of the enforced O $^\alpha$ -O $^\beta$ homolysis in aqueous solution, we can indeed observe these trends by plotting the average number of hydronium ions in the solvent versus the reaction coordinate $R_{\rm {OO}}$ (see circles in the lower graph in figure 6.5 and the dotted lines to guide the eye). The water ligand O-H distances $R_{\rm {OH}}$ were taken as the order parameters: all 10 $R_{\rm {OH}}<1.3$ Å means that no hydrolysis has taken place. At the reactant side (small $R_{\rm {OO}}$ ), hydrolysis is rarely observed during the 2 ps simulations and only the one hydronium ion which we started with (originating from the hydrogen peroxide when it reacted with iron(III), see previous sections) brings the average number to 1 H

. Going towards higher $R_{\rm {OO}}$ values, the oxidation state of the iron ion goes to four and the complex is seen to become more acidic, confirming the second trend mentioned. At $R_{\rm {OO}}=2$ Å, 62 % of the time a (second) proton was donated to the solvent by the complex (in the 1.3 ps before H abstraction by the leaving O $^\beta$ H. from a solvent water), which justifies the previous comparison of $R_{\rm {FeO}}$ with that of the hydrolyzed ferryl ion ([Fe $^{\rm {IV}}$ O(OH)]

) in the upper graph. At $R_{\rm {OO}}=1.6$ Å, the average number of 1.96 hydronium ions seems out of order in this trend. In the simulations, we see that for this run the two hydronium ions are most of the time jumping freely around in the solvent. In the other runs however, we find that most of the time one of the protons jumps back and forth between the ligand and a solvent water molecule and thus remains in the neighborhood of the complex. Apparently we can separate the ligand hydrolysis into two stages which show resemblance with the dynamics of free hydronium ion transfer in water (cf. ref. TLSP2), namely: 1) a fast process which involves the sharing of the proton by a ligand and a solvent molecule (or two solvent waters for the free hydronium ion, with a frequency $\nu \approx$ 5 ps $^{-1}$ ) and 2) a much slower process, which is connected to the actual stepwise diffusion of the hydronium ion through the solvent. The latter process concerns changes in the second coordination shell hydrogen bond network which in water was found to have a frequency of about 0.5 ps $^{-1}$ .[53] Obviously, our 2 ps AIMD simulations are too short to capture good statistics of the slow process, so that in each simulation we either see the excess proton being shared by two water molecules in the solvent (namely in the run with $R_{\rm {OO}}=1.6$ Å,) or it is being shared by a ligand and a solvent molecule (as in all other runs). Fortunately, already from the distribution in the fast jumping process we obtain information on the acidity (i.e. the ability to donate a proton to the aqueous environment) of the iron complex, as shown in figure 6.5, but for comparison with experimental p

values we need to include also the slower hydronium ion transport. The run with $R_{\rm {OO}}=1.6$ Å confirms the first trend mentioned in this paragraph: replacement of a water ligand by a hydroxo ligand facilitates the oxygen-oxygen homolysis. In our constrained MD exercise this is seen by the lower absolute constraint force resulting in a dent in the mean constraint force profile in figure 6.4 and also in the $R_{\rm {FeO}}$ profile in figure 6.5. If we could afford better statistics by performing much longer simulations, in principle the two states (pentaaqua versus hydrolyzed tetraaqua hydroxo complex) would be sampled with correct weights, giving the correct mean force of constraint and free energy profile. In our result however, we find for all runs except the one with $R_{\rm {OO}}=1.6$ Å mostly the pentaaqua complex, so that we should take into account an overestimation of a few kcal/mol for the free energy barrier. Moreover, if we would be interested in calculating the reaction rate of the O-O homolysis reaction in water we should either control the hydrolysis process by including it in the reaction coordinate or we should expect a large deviation from the transition state theory reaction rate, and therefore perform the cumbersome computation of the transmission coefficient in the pre-exponential factor. We can nevertheless conclude that our estimation of the free energy barrier of the O-O homolysis of the iron(III)hydroperoxo intermediate in aqueous solution indicates that this formation of a ferryl ion and the OH. radical is a likely second step in Fenton-like chemistry. And secondly, the simulations confirm the hypothesis that water ligand hydrolysis plays an important role in the process.